
Well, this is also 45 degrees, so the turn we need to make here is also 180 minus 45, or 135 degrees. Let's put our original triangle back in place and now we want to figure out how much of a turn we need to make on this side. So if we watch this turtle when I hit return and we go forward, we should go all the way to the other corner. And since a hectostep is 100 steps, that means we want to go forward 100 times the square root of 2. So what does that mean about the length of this line? The length of this line times the length of this line is 2 hectosteps, So that means that one of these lines is the square root of two hectosteps. OK, we know the area of this square is two hectosteps, because the area of this is one hectostep, the area of this square is one hectostep squared, so this has to be two hectosteps squared. Now let's rearrange these pieces for a minute. And these two squares make up two square hectosteps. So if each side is 1, then the area as a whole is 1 hectostep. So this is one hectostep, so this square is one by one. But, we said this is 100 steps, actually let's make up our own unit, we'll say this is one hectostep, OK, a hectometer is 100 meters, a hectostep is 100 steps. Um, let's consider for a minute, what we're gonna have to look at is, well we're gonna start with two squares here, and think for a minute about the area of squares, and then it'll be clear in a minute why we have to worry about the areas.
Now we have to figure out the length of this line right here, and that's a little bit trickier. So let's turn right 135 degrees and see how that looks. So the total amount of turn, the angle in this whole sector here is 180 minus 45, or 135 degrees. And we know that this little blue part here, this little angle is 45 degrees. Let's look here at the turn, OK? Now in general, when we're turn a corner, you see we're still facing in this direction, this, this entire angle, if we take the blue corner of the triangle together with this little sector is 180 degrees. How much of a turn do we have to make? How much of a turn does the turtle have to make? So we know that this angle is 45 degrees. If we cut that angle in half, we have 45 degrees, right here.
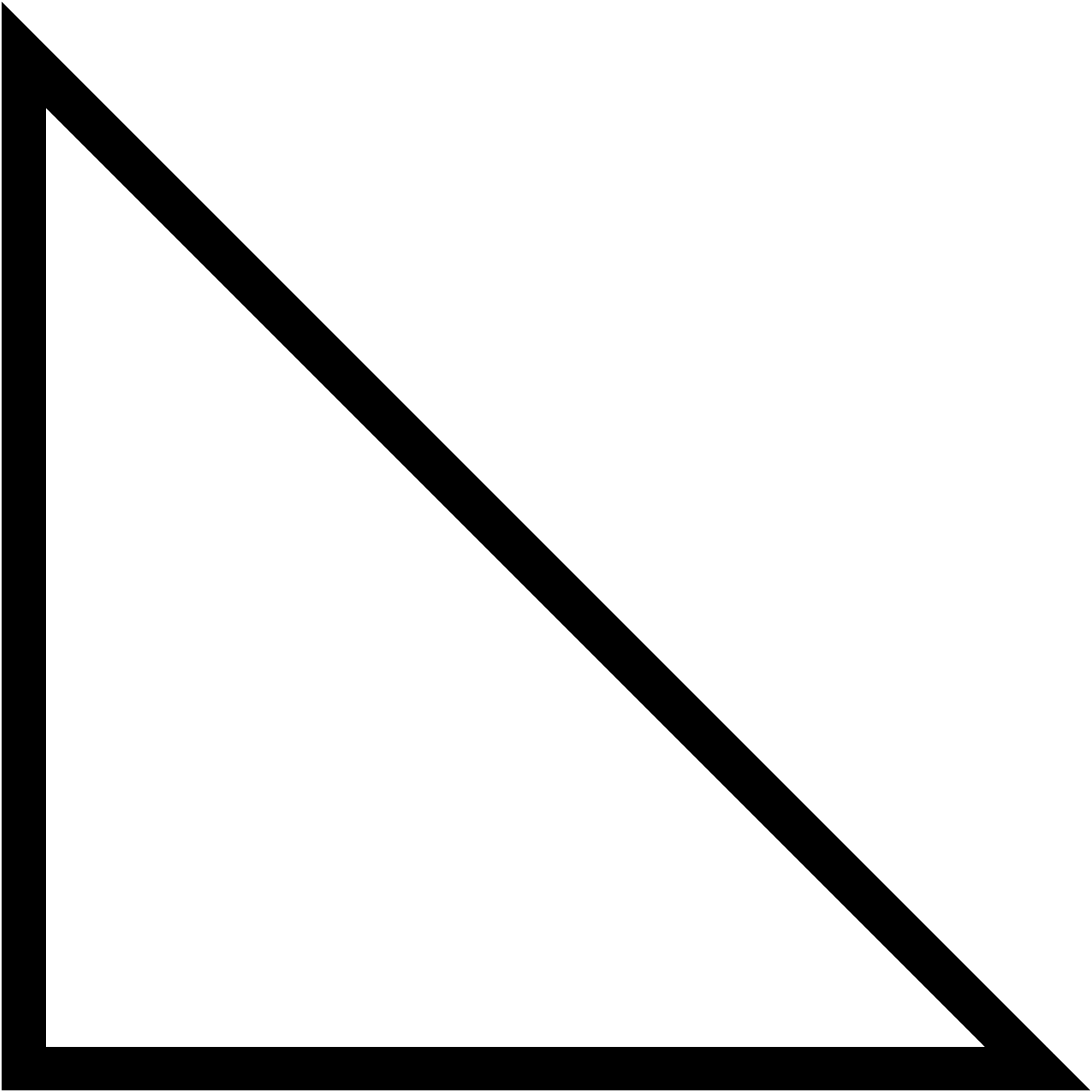
We know that each corner of the square is 90 degrees. Well, this diagonal cuts this angle right in half, doesn't it? If we look at this square, we see that this triangle is made by starting with a square and drawing the diagonal. Well, let's consider for a minute how we make a right angle isosceles triangle. Now I want to figure out how much of a turn I have to make here. So, the start is easy, I can just say forward 100, and that takes me up to the corner. So, um I happen to know that this particular triangle is 100 steps on this side, and 100 on this side.

Let's put our turtle right over here, and for now I'm not gonna draw anything on the screen, I just want to see if I can get the turtle to follow the path of this edge. This triangle is isosceles because these two sides are the same length, and it's a right triangle because we have a right angle right here.
#Right isosceles triangle how to
You can't haveĭifferent side lengths, or you couldn't have differentĪngles right over here and also meet those conditions.OK, so now we're gonna consider how to draw an isosceles right triangle using the turtle. So this is the only side thatĬan connect those two points. Point are going to be there are no matter what. Two lengths constant, then this point and this Rephrasing that, is this the only triangle Now they say, is thereĪ unique triangle that satisfies this condition? So another way of So it seems like we've metĪll of our constraints. This side have length 3, so 3 and then 3 right over there. Length 3, and it's got to be a right triangle. Isosceles triangle, so that means it has to haveĪt least two sides equal and has two sides of length 3.


 0 kommentar(er)
0 kommentar(er)
